During a visit to Bolivia in 2001, the sight of marine sedimentary strata high in the Andes led to the consideration of the magnitude and direction of the tremendous forces involved. They are considered to have caused several major events such as the unrelenting unidirectional movement of the South American plate away from Pangea, formation of the Cordilleras by compression and the lifting of the oceanic crust and sediments from below sea level to five kilometers above it.
From an engineering perspective, however, the driving forces associated with heated convection currents as per the accepted model of Hess (1962) at convergent plate margins appeared to be neither large enough nor sustainable over geological time spans of say 150 Ma to cause this level of orogenic activity. This article offers an alternative analysis based on the forces associated with the rotational speed of the Earth to explain tectonic plate movements and orogenic processes.
Current thinking is that the movement of a continental plate (CP) is the result of the ‘pulling action’ applied to it by the subduction of the higher density oceanic lithosphere (OL) as it descends below the CP. The direction of the heated convection currents considered to cause subduction must vary over time and distance. Thus, it is difficult to reconcile unidirectional tectonic plate movements breaking up a supercontinent such as Pangea with omnidirectional changing forces due to convection currents.
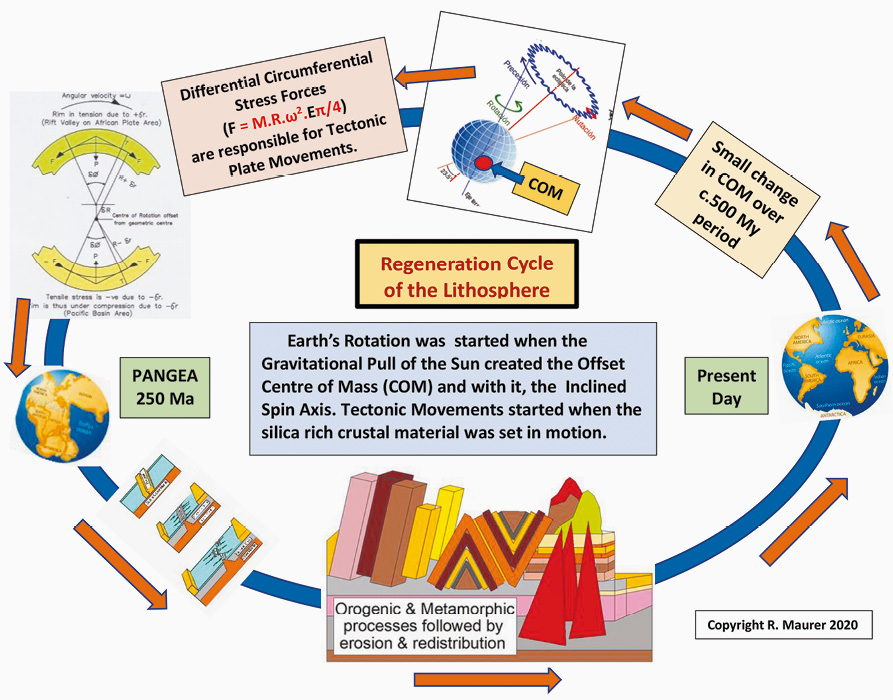
Analysis of the forces generated by the rotational velocity of the Earth (Maurer, 2020) suggests a quite different mechanism to explain the currently accepted 500-million-year cyclical break-up and reassembly of supercontinents.
Figure 1: Principal forces and vectors. Credit: Robert Maurer.
Earth’s Center of Mass Offset
It was noted that the ‘wobbling’ Earth, with its change in the orientation of the inclined spin axis, the associated Milankovitch Cycles, the nutation (nodding motion) as well as the variable and cyclical ‘Chandler Wobble’, closely mimics that of an unbalanced rotating shaft whose Center of Mass (COM) is offset from its spin axis. An everyday example is the vibration of an unbalanced wheel on a motor vehicle.
Illustrations of the break-up and dispersal of Pangea since the Jurassic period show that the South American plate moved west while the Australian plate moved east relative to the essentially central position of the African plate. The Indian plate also moved east before turning north-east. At the same time, the Laurasian plate moved north, later splitting into the Eurasian and North American plates with the opening of the North Atlantic. These movements suggest that the major plates were being redistributed from the heavier side of the Earth, occupied by Pangea, to the lighter side, occupied by the proto-Pacific. This premise was reinforced by an 8 km difference in mean elevation between the surface of the African plate and the floor of the Pacific Basin. Using a simple algebraic equation, based on isostatic equilibrium to estimate the COM or fulcrum point, and taking the elevation difference into account, the calculation showed that the COM was 2.09 km off-center in the African hemisphere.
However, the simple introduction of a displaced or offset COM will only describe the rotating Earth as an unbalanced rotating flywheel. As such it does not describe the circumferential forces (linked to tectonic movements) which allow the Earth’s rigid lithosphere to move relative to the mantle.
To quantify the forces associated with the offset COM, it was necessary to mathematically model the Earth as a rotating unbalanced body. Kepler’s Laws of Planetary Motion (published between 1609 and 1619) relating to the elliptical orbit of the Earth around the Sun showed that the Earth’s orbital velocity increased as it approached perihelion (the point in the orbit at which a planet is closest to the Sun) and decreased by the same amount on its way toward aphelion (furthest from the Sun). In this respect the planets can be seen as rotating about their axes in a stable controlled position. As such the mathematical model is given a high degree of validity.
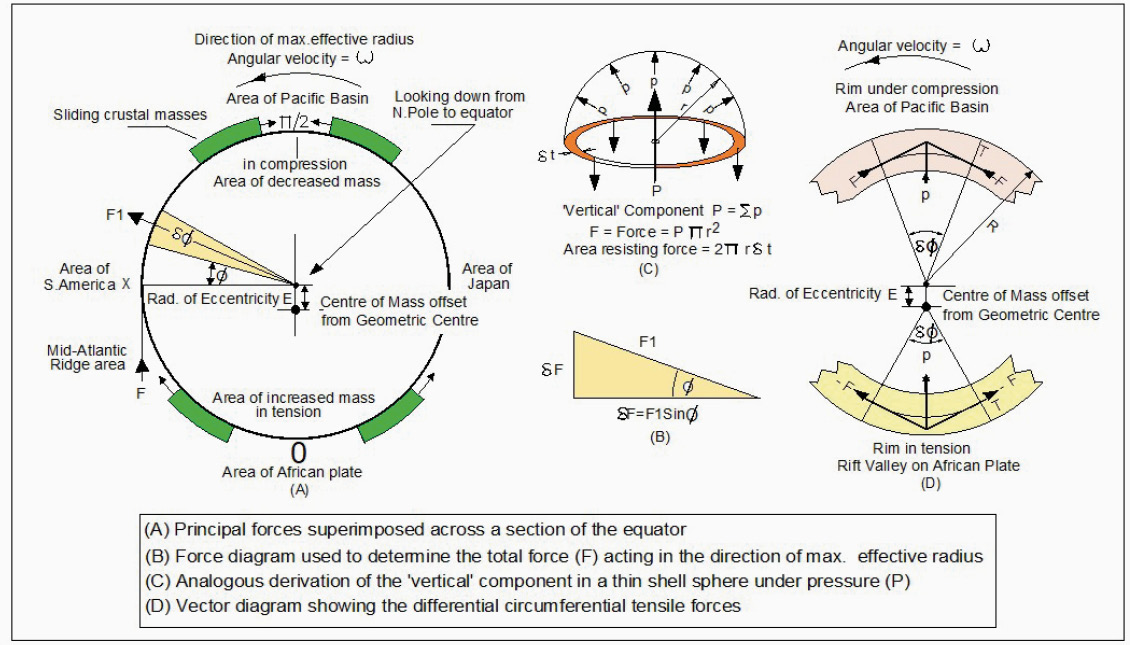
Circumferential Tensile Forces
By approaching the problem in terms of a thin shell moving relative to the mantle, it is possible to consider which increments of the tensile force are responsible for putting the Pacific Basin under compression and the African plate under tension. The African Rift Valley is today a case in point.
In calculating the effects of the circumferential tensile forces (Fcir) at the Earth’s surface due to the COM being offset from the spin axis or geometric center, the term ‘Radius of Eccentricity’ (E) is introduced to denote the magnitude of the offset. Figure 1 shows this approach as a vector diagram in which the differential circumferential stresses are annotated. To simplify discussion and further calculations, E was placed at 1 km offset from the spin axis. Seen in context, this equates to (1/6400) × 100 or 0.016% of the Earth’s mean radius of 6,400 km. The magnitude of the derived differential circumferential stress (Fcir) will thus be dependent on E. In a limiting case, if E=0, the differential stress will be zero, as the Earth will be balanced. The derived equation Fcir = MRω2Eπ/4 relates Fcir to E, where R is the mean radius of the Earth, ω its angular velocity and M is the mass of a segment of the crust 1,000m × 1m × 1m. Using averaged published data for M, R and ω, sourced from USGS and National Geographic Society publications, the stress value is calculated to be 0.074 Newtons/mm2 (10.8 lb/in2). To understand a stress value of 0.074 N/mm2, this figure equates to 118 people each weighing 81.8 kg (180 lb) pushing a braked motor vehicle with a rear surface area of 1.3 m2.
Figure 2: Separation of tectonic movements from subduction.
Splitting Supercontinents Apart
In trying to establish that the differential circumferential forces (Fcir) are sufficient to split supercontinents apart, it should be borne in mind that the tensile strength of the oceanic lithosphere (OL) varies considerably with age and depth. The older OL (>10 Ma) is mechanically able to withstand lithospheric forces such as tectonic interactions, whilst the younger OL is considerably thinner and whose mechanical strength is substantially reduced by heat interactions (Cadio and Korenga, 2016). As such, rifting and ridge formation are more likely to occur in the younger OL (Lynch and Morgan, 1987). Based on this
premise the rift that opens within a supercontinent will be along the weakest lines that allow separation to occur and magma to readily extrude onto the sea floor. The lack of distortion of the paleomagnetic lines demonstrates the lack of any viable compressive forces involved. On this basis the rift that opens within a supercontinent will be continuously pulled apart to allow the extrusion of magma onto the sea floor. The widening of the new ocean will be by sea floor stretching as opposed to sea-floor spreading. Thus, a ridge-push force exerted by rising magma can be zero so far as tectonic plate movement is concerned.
Consideration of Momentum and the Subduction Cycle
Perhaps the most important aspect in the breaking up of the supercontinent Pangea is that the plates will accelerate from almost zero velocity (Vo) to the present-day velocity (Vcp) of about 11 mm/year. In doing so the CP has momentum imparted to it as per Newton’s Second Law of Motion, which is Momentum = Mass × Velocity, as V increases from Zero to Vcp, the equation will be:
Mcp (Mass of CP) * Velocity (Vcp-Vo) = Mcp*Vcp.
Whilst the velocity is low, the continental mass is extremely large. The overall momentum will make the slow but relentless movement of the CP unstoppable until it meets another CP. India crashing into the Eurasian plate creating the Himalayas is a case in point.
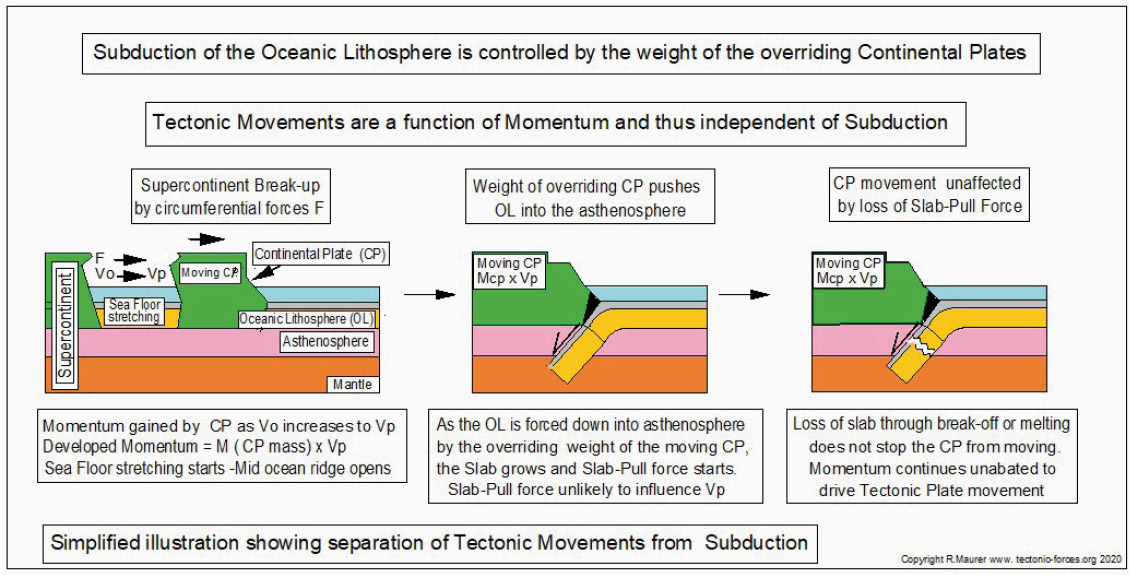
As the CP is forced away from the supercontinent (SC) by the circumferential forces, the weight of the CP will push the oceanic lithosphere under it into the asthenosphere (i.e., the ductile upper mantle) and finally into the lower mantle (Figure 2). Seismic observations have shown that a ‘knee bend’ forms in the oceanic lithosphere (OL) as it bends downwards as a ‘slab’ into the asthenosphere. The force associated with the weight of the slab is currently credited as being the major force aiding subduction and tectonic plate movements. The resistive forces associated with slab-pull are essentially friction and viscosity. Examination of the major forces presently considered responsible for subduction can be summarized as follows:
Owing to the density difference,
Subduction Force = Net Slab-Pull Force + (gravitational force due the weight of the CP (FMcp) above the OL) + circumferential forces acting on the CP – Bending stress of the OL (FBol) + the upward buoyancy force (Fby).
The upward buoyancy force may be considered as the force needed to sustain isostatic equilibrium. The ridge-push force has already been shown to be negligible.
Separation of Subduction from Tectonic Plate Movement
The introduction of momentum and circumferential forces is new to the study of subduction. This innovative introduction has thrown up some interesting and far-reaching conclusions. The inevitable loss of the slab and thus the slab-pull force, either by detachment or by partial melting in the asthenosphere, has not hindered the movement of the CP. The equations for subduction are now,
Subduction Force= Resultant (FMcp + Fcir) > FBol + Fby
The momentum of the continental masses will keep them in motion.
The logical and unexpected conclusion is that continental plate movement is independent of slab-pull and subduction. The separation of subduction from tectonic plate movements is a major change in the study of plate tectonics. Subduction is now seen as being a consequence of tectonic plate movements rather than the driving force. This has brought with it the observation that slab-pull is applicable to the tensile stressing of the OL and possibly the ridge-push forces that may now be considered as ‘ridge-pull’ ones. The origin of these forces associated with rifting and ocean ridges is still seriously debated.
Birth of Tectonic Plate Movements
It is interesting to note that all the planets (except Venus – considered as being upside down) rotate on their axes with the same anticlockwise motion as the Sun. The terrestrial planets have an almost identical polar/equatorial diameter ratio, but the gaseous planets are more oblate. Apart from Mercury and Venus, the planets’ Figure 3: Earth’s continuous regeneration cycle. The break-up of Pangea is given as an example. rotational periods are within the range 10 to 24 hours, and have the same bottom-left to top-right inclined spin axis (except for Venus and Uranus). The uniformity of planet behavior is puzzling as the gravitational pull of the Sun alone does not yield a satisfactory explanation regarding the rotation of the planets. Current wisdom favors the concept that the angular momentum of the low angular velocity swirling gas cloud during the formation of our galaxy, is now being shared between the Sun and the planets rotating at higher angular velocities.
Figure 3: Earth’s continuous regeneration cycle. The break-up of Pangea is given as an example. Credit: Robert Maurer.
As it is impossible to rotate a body about its dimensionless center line, it is likely that the COM offset was created when the Sun pulled on the embryonic partially cooled planets, towards the end of their accretionary stage, to cause them to rotate in the same direction as the Sun. The angular momentum imparted to the larger accretionary masses would ensure their permanent rotational mode. This approach also gives a plausible explanation regarding the creation of the tilted N–S spin axis of a planet.
