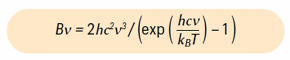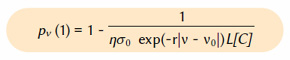Recent advances in climate change research: Part XIV – The doom of a photon on a random walk

Recent Advances in Climate Change Research: Part XIV – The Doom of a Photon on a Random Walk

Should I stay or should I go now?
If I go there will be trouble
And if I stay it will be double
So come on and let me know
– The Clash (1982)

The history of an individual infrared photon being emitted upwards from Earth’s surface into a static atmosphere is analysed as a one-dimensional random walk. Will it return to Earth, or depart into outer space, or will it stay in the atmosphere forever? The photon’s frequency and the CO₂ concentration in the atmosphere determine its fate. The random walk applied to the radiation of photons lacks essential physics but provides a simple educational model which illustrates how absorption, followed by re-emission, result in a net stream or flux of photons back to Earth’s surface.

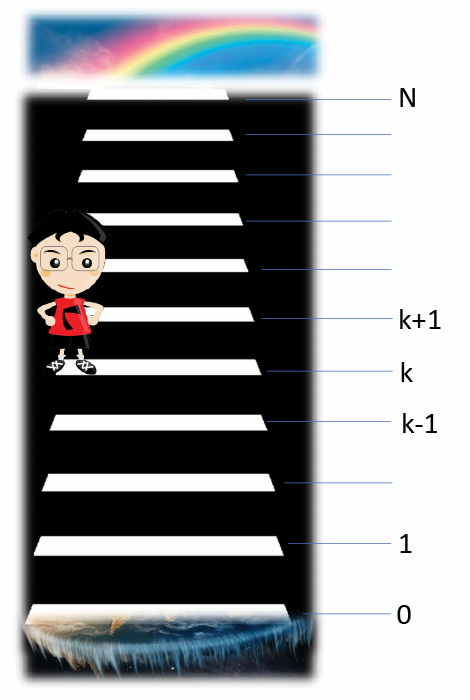
One day when Brigitte is climbing the Haiku Stairs she meets Phôs. Brigitte, let me know, Phôs says. I’ve been walking the stairs for some time now. I am born from Gaia (Earth) but she destroys me if I fall onto her. I cannot go to the top since Rainbow then reaches down on me to carry me up to space. Should I stay, or should I start a random walk, in the hope that I will walk the steps forever? Brigitte says, if you go there will be trouble. The random walk will be your doom.

The random walk means that you hop one step up or one step down with equal probability, independent of the direction of all your previous hops. Suppose that Haiku Stairs has N+1 steps. The first step 0 is close to Gaia and the top step N is close to Rainbow. We are now at step k. What you’re asking me is your chance of avoiding fatality – extinguished by Gaia at step 0 or captured by Rainbow at step N.

Let p(k) be the probability that you hop to the bottom step where you are extinguished by Gaia, given that you start at step k. In two special cases, the value of p(k) is easy to determine. If you start at 0, you fall onto Gaia and you are extinguished immediately, so that p(0)=1. On the other hand, if you start at step N, you’re captured by Rainbow, who will never more let you hop the stairs, so p(N)=0.

When Phôs starts at k (0<k<N), Brigitte breaks her analysis of Phôs’s fate into two cases based on the direction of his first hop:

If his first hop is down, then he lands at step k-1 and eventually he falls onto Gaia with probability p(k-1).

If his first hop is up, then he lands at step k+1 and he eventually falls onto Gaia with probability p(k+1).

By the law of total probability which expresses the total probability of an outcome which can be realised via several distinct events, Brigitte expresses the probability of Phôs falling onto Gaia as: p(k) = (1/2) p(k-1) + (1/2) p(k+1), p(0) = 1, p(N) = 0

In mathematics, this just a linear recurrence: p(k+1) = 2p(k) – p(k-1)

with well-established methods for solution. High school students who are familiar with finite differences (see GEO ExPro Vol. 15, No. 2) are also on familiar territory by writing the recurrence equation in the form: p(k+1) – 2p(k) + p(k–1) = 0

The left-hand side is seen as the finite difference (with step one) of a second derivative.

The right-hand side dictates that the second derivative be zero; therefore, the solution for p(k) must be a linear function p(k)=a+bk where the constants a and b are determined by the boundary conditions p(0)=1 and p(N)=0. Brigitte tells Phôs the probability: p(k) = 1-k/N [1]

She continues: Phôs, when you start at step k in a stair with N+1 steps, then you’re destroyed by Gaia with probability 1-k/N. Moreover, it is straightforward to calculate the probability that you’re captured by Rainbow. Leaving it as an exercise, the answer is 1-p(k)=k/N.

This is bad news for you, Phôs, Brigitte concludes. The probability that you end your fate at the bottom or on the top of the stairs is 1-k/N+k/N=1. There is zero probability that you’ll hop the stairs happily forever.

Phôs asks Brigitte, what is my destiny if the number of steps in Haiku Stairs is infinite? Brigitte lets N tend towards infinity in the probability equation [1] and she finds 1. Even for an infinite number of steps, you are extinguished by Gaia with probability 1. This is true no matter where you start.

Phôs in the Atmosphere

Before reading on, the interested reader may want to consult ‘Part XI: How Earth’s IR Photons are Transferred in the Atmosphere in the Presence of CO₂‘ (GEO ExPro Vol. 18, No. 1). The reader may also consult Wilson and Gea-Banacloche (2012) who have discussed, in a similar way to our attempt, the history of an individual photon when treated as a random walk, consisting of absorption and re-emission events by CO₂ molecules in a hypothetical static atmosphere.

Following the theory of Part XI, we consider the exponential atmosphere whose composition is assumed to be the function of height only. In this atmosphere, the transfer function taking the photon from the surface of the Earth to height z is Qν(z,0)≈exp(-z/lν), where lν=1/nσν is the mean free path or the mean distance travelled by a photon before being absorbed. Here, n is the local CO₂ density and σν is the CO₂ cross-section which is a measure for the probability of absorption, or the ability of a CO₂ molecule to absorb a photon of a particular frequency or wavenumber. The cross-section can be approximated by the function σν=σ0 exp(-r|v-v₀|), where σ₀=3.71·10^-19 cm², r=0.089 cm and v₀=667.5 cm^-1, close to the approximation suggested by Wilson and Gea-Banacloche (2012). Air has 2.5470·1019 molecules/cm³. Then, when the CO₂ concentration is given in ppm by [C], the local density close to Earth’s surface is n=η[C] molecules/cm³ where η=2.5470·10¹³.

After being absorbed, the photon is re-emitted, with a 50/50% probability of being sent upwards in the atmosphere or downwards, back to the Earth. If it goes upwards, then it travels another distance lν before it is absorbed again. The story of absorption and re-emission continues in this way. The question is what is the probability that the photon will eventually get back to Earth, where it heats Earth’s surface and ends its life? Alternatively, we may ask what is the probability that it eventually escapes into space?

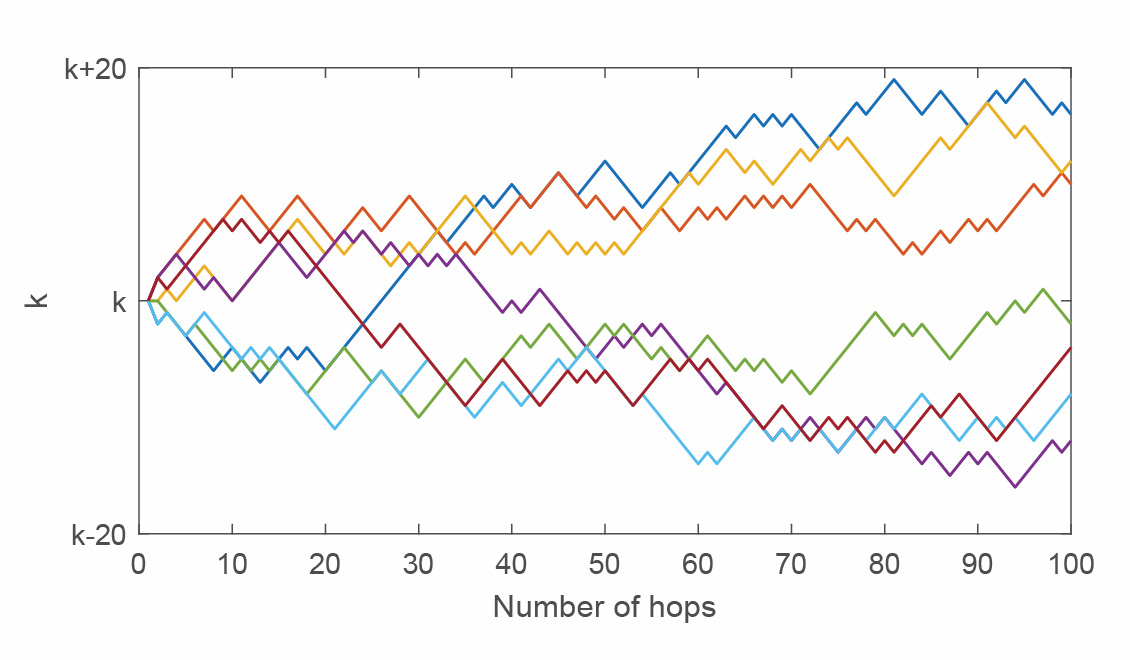
Let’s use the simple random walk formula [1] to study the fate of a photon emitted upwards by the Earth’s surface at ‘step 0’. Since the photon has travelled one mean distance, it is on ‘step 1’ when it starts on the random walk, with probability of return: p(1) = 1-1/N

To evaluate this probability, we need an estimate of the number of steps or ‘layers’ N of the atmosphere. The exponential atmosphere is characterised by the scale height L=8,000m. In the exponential atmosphere, for every L rise in altitude, the density and pressure of air drop by a factor e=2.7; thus, L provides a measure of the thickness of the atmosphere. Therefore, we may take the number of layers to be: N = L/lν = ησν L[C]

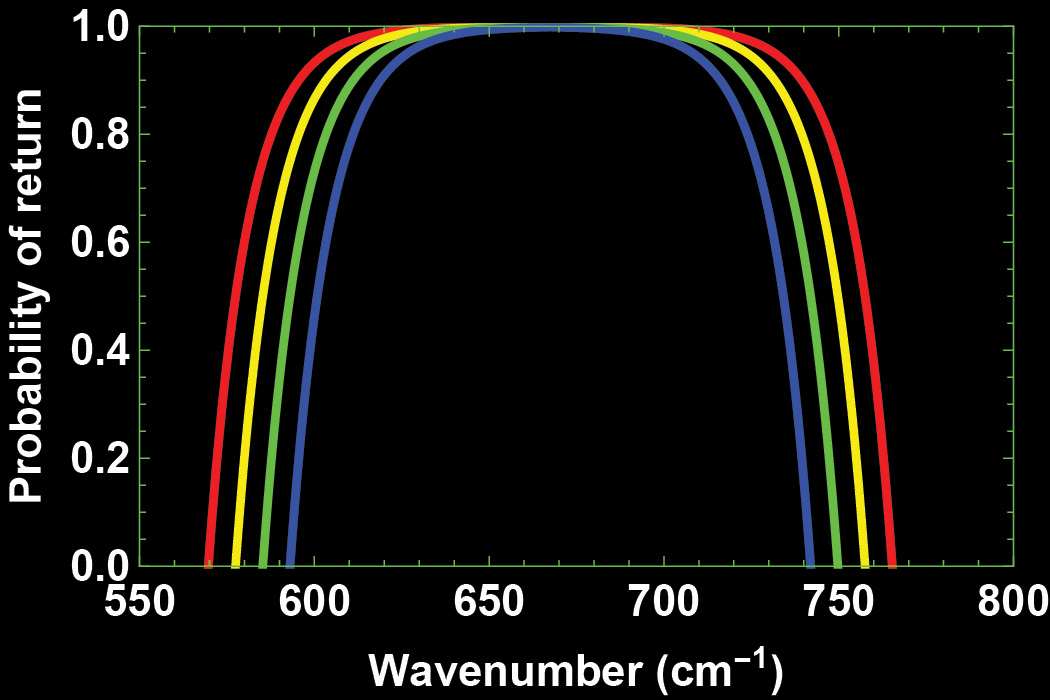
Let’s estimate N by supposing that the photon is at the centre of the absorption band at wavenumber ν₀=667.5 cm^-1, when being emitted upwards from the surface of the Earth. Assume that [C]=400 ppm. The mean free path becomes 2.646m so that N=3024. The probability of returning to the Earth becomes p(1)=0.9997. A photon with frequency or wavenumber near the centre of the absorption band is virtually certain to make the return to Earth. If the CO₂ concentration is doubled, the probability doesn’t change much; it becomes p(1)=0.9998. Any CO₂ increase has minor effect on the photons in the center of the absorption band. This part of the band is ‘saturated’.

Suppose instead that the photon leaves Earth’s surface away from the centre of the band, say at its wings at wavenumbers ν=585.2 or 749.8 cm^-1 where σν has fallen off by several orders of magnitude. Now, the mean free path is 4,014m, the number of layers in the atmosphere is 3 (N=2) and the probability of returning to the surface is p(1)=1/2. The probability then for the photon to disappear into outer space is 1-p(1)=1/2.

No surprise, actually. It is a 50/50 chance to end at step 0 or step 2 when the photon starts at step 1. However, if the CO₂ concentration doubles, these numbers change considerably: p(1)=3/4 and 1-p(1)=1/4. Hence, when the CO₂ concentration doubles, the probability of the photon at the two selected frequencies at the wings of the CO₂ cross-section coming back to Earth increases by 50%, and the probability to end in outer space is halved.

Clearly, because of the exponential decay of the CO₂ cross-section, the effect of a CO₂ increase is important mostly around the wings of the CO₂ cross-section. In addition, we observe that the mean free path of the photon in our simple model is halved by a doubling in CO₂ concentration. This implies that the number of layers in the atmosphere doubles, so that a large increase in the CO₂ concentration widens the range of photon frequencies that are blocked from reaching outer space.

Number of Photons Emitted from Earth’s Surface

In ‘Part VIII: How CO₂ Absorbs Earth’s IR Radiation‘ (GEO ExPro Vol. 19, No. 3) we displayed the number of emitted photons from Earth’s surface as a function of wavenumber for the surface temperature of 288K (15°C). How do we calculate the number of photons? We start with the Planck function:

which tells the emitted power per unit area per steradian per wavenumber interval. Here, h and kB are the Planck and Boltzmann constants and c is the speed of light. We now remind the reader that the energy flux (the Stefan–Boltzmann law) is given by the famous formula: F = π ∫₀^∞dν Bν = σ_B T⁴ 

It tells that a blackbody source of temperature T radiates a total power (per area per steradian) across the entire electromagnetic spectrum of σ_B T⁴/π where σ_B is the Stefan–Boltzmann constant.

The photon has energy hcν. Introducing the photon density Nν=Bν/hcν the photon flux NP is given by: NP = π ∫₀^∞dν N_ν = σ_H T³

where we name σ_H = 4πkB³ ζ(3)/(h³c²) = 1.5205·1015 photons m^-2 s^-1 K^-3 the Haas constant, where ζ is the Riemann zeta function, which has ζ(3) ≈ 1.202.

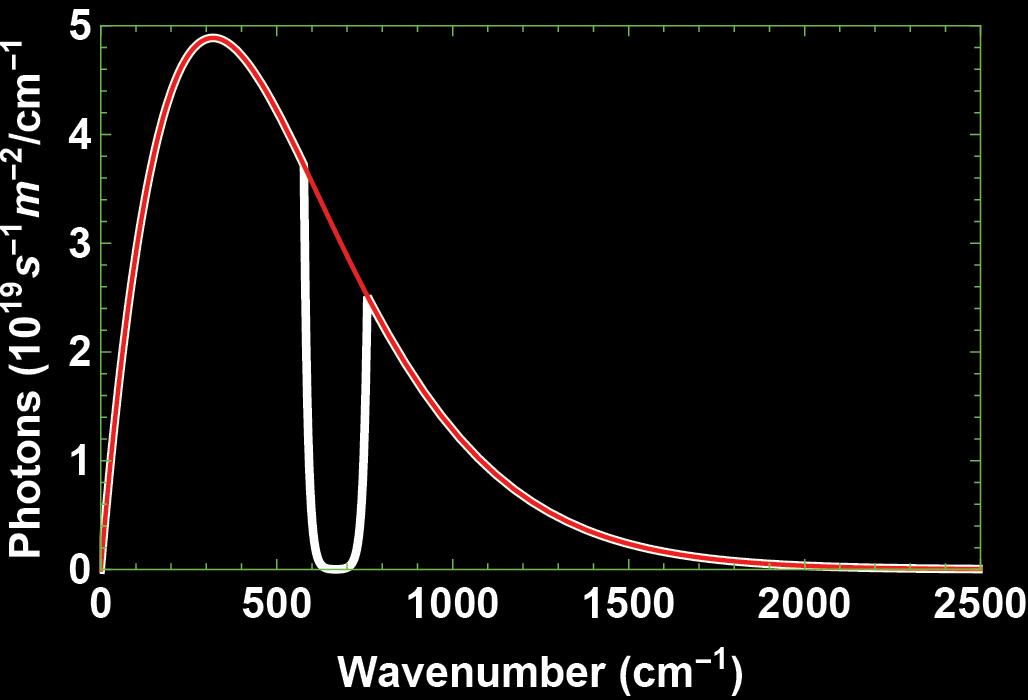
The total number of photons emitted by a blackbody in thermodynamical equilibrium thus is close to 1.5·10¹⁵·T³ photons per s per m². This number was first calculated by Haas (1938). For Earth’s surface temperature of 288K (15°C) the total number of photons emitted is 3.63·10²² photons per s per m².

Number of Photons Returning to Earth

The number of photons that return to the surface of the Earth can be estimated by use of the random walk probability formula. Earth’s emitted photon flux depends only on the Earth’s surface temperature. To get the flux of return we may multiply the emitted flux by the probability of return:

where the ν-subscript denotes that the probability depends on the wavenumber. This probability is plotted in Figure 4 for four different CO₂ concentrations. It is close to one near the centre of the CO₂ cross-section around ν₀ but drops towards zero at the wings of the cross-section. Due to the exponential decay of the cross-section the probability pν will become negative at wavenumbers ν_±=ν₀±ln(ησ₀ L[C])/r and we replace all negative probabilities by zero. The total number of photons returning then can be written: N_Pⁱⁿ = π ∫_v-^v+ dν N_ν p_ν (1)

Numerical integration of the above equation gives the number of photons returning to Earth’s surface: 4.9×10²¹ photons per s per m². This is 13.5% of those that the Earth initially emitted.

Number of Photons Leaving for Outer Space

In Figure 5 (the white line) we display the number of photons that are departing for outer space per second per square metre per wavenumber interval. The total number of photons leaving the atmosphere is the total number of photons emitted by Earth minus those returning to Earth’s surface. The percentage is 86.5%.

Warming Effect by CO₂ Doubling

The Earth is considered a blackbody in the infrared spectrum. It radiates F=σ_BT₄ =390 W/m². In the same way that we calculated the number of photons returning to Earth’s surface we can calculate the inward flux to Earth for a given CO₂ concentration. First, multiply the blackbody radiation per wavenumber by the probability of photon return; second, integrate numerically over all wavenumbers.

The monthly mean CO₂ content is measured at the Mauna Loa Observatory in Hawaii. At the end of November 2021 the concentration was measured as 415 ppm. By calculating the inward radiation fluxes from this time to the time a doubling is achieved, we find the fluxes 64.8 W/m² and 71.1 W/m², giving the change in flux ΔF = 6.3 W/m². This simple calculation assumes that the temperature profile of the Earth system does not change during the increase in CO₂.

Due to the simplicity of the model, the inward radiation flux numbers cannot be trusted. However, in the absence of processes other than the ones discussed so far, the model shows that the absorptive strength of CO₂ would be sufficient by itself, to cause an increase in the inward flux.

The Effect of CO₂ Radiation Efficiency on Warming

We speculate if the model can be made slightly more realistic by including the CO₂ photon radiation efficiency. The random walk model assumes that every CO₂ molecule that absorbs a photon also radiates the same photon, in a random direction. However, in ‘Part IX: How CO₂ Emits IR Photons‘ (GEO ExPro Vol. 17, No. 4) we showed that only around 5% of the CO₂ molecules after having absorbed a photon radiate a photon. The rest of the CO₂ molecules relax by colliding with N₂ and O₂ molecules.

By including this effect, which we may call the CO₂ radiation efficiency, we redo the calculations and find that the inward fluxes are 37.4 W/m² and 43.8 W/m2. Although the change in flux is the same, ΔF=6.3 W/m², the inward fluxes in Earth’s energy budget are significantly reduced due to the low radiation efficiency (5%) of the CO₂ molecules in the atmosphere. The inward fluxes are reduced by around 40% by a 95% reduction in CO₂ radiation efficiency.

Conclusion

The model of random walk applied to the radiation of photons is simple but lacks obviously essential physics. Nevertheless, the mathematics of the model provides a simple picture to illustrate how absorption, followed by re-emission, result in a net stream or flux of photons back to Earth, at those frequencies where the atmosphere is strongly absorptive, that is, where the CO₂ cross-section is large, implying that the mean free path is short, so that the number of atmospheric layers is large. Absorption and re-emission of photons in random directions prevent some photons escaping out to space. They are returned to the surface of the planet where they heat Earth. At the wings of the CO₂ cross-section, the mean free path is much larger, giving the photons at those frequencies a higher probability of departing Earth into outer space.