‘An idea, like a ghost, must be spoken to a little before it will explain itself.’ – Charles Dickens
Dickens’ creative genius was praised by fellow writers, and this quote shows he was interested in the creative process.
You might have a great idea, but without ‘talking to’ or working with it, it will never be realized. An idea may come out of nowhere but you have to do some deep investigations before it can be explained and utilized fully. In other words, you never know how it will turn out unless you work hard with it first.
130 years after Dickens, a top-notch scientist was sitting in ‘The Tent’, Schlumberger’s research building in Cambridgeshire. The building is a theater of exciting technology, providing a stimulating intellectual environment for world-class scientists. It is recognized for its active geophysics department working on a wide range of theoretical and experimental research, with seismic as the common thread. The scientist is studying how hydrophone and geophone measurements can be combined into deghosting, the basis for broadband seismic. He realizes that only the vertical component of the velocity measurement is used in the deghosting process, and deghosting at the time is only applied on a streamer by streamer basis, in 2D. Is there any use for the two other components of the velocity measurement, he wonders?
This example compares images of intricate Paleogene sand injectites, which are commonly associated with reservoir units in the North Sea. The IsoMetrix technology resolves these complex structures in all directions, unlike the hydrophone-only conventional equivalent. (Source: WesternGeco)
This example compares images of intricate Paleogene sand injectites, which are commonly associated with reservoir units in the North Sea. The IsoMetrix technology resolves these complex structures in all directions, unlike the hydrophone-only conventional equivalent. (Source: WesternGeco)
Using Crossline Data
Let’s go back to basics. The acoustic wave equation governs the propagation of acoustic waves through the Earth and the water layer where the streamers are towed. It describes the evolution of acoustic pressure P or particle velocity vector V as a function of position and time. To arrive at the wave equation, physicists make use of conservation of mass and Newton’s law, which tells us that the acceleration of particle motion (the rate of change of velocity, ∂V/∂t) is related to the gradient of the pressure wavefield (P). For each of the components, it yields:
ρ ∂Vx /∂t = -∂P/∂x
ρ ∂Vy /∂t = -∂P/∂y
ρ ∂Vz /∂t = -∂P/∂z
where ρ is the density of water.
As mentioned above, Vz is used together with P in 2D deghosting. If x denotes the inline, streamer direction, there is no direct value of any Vx measurement since the hydrophone spacing is so dense for point-receiver recording that ∂P/∂x can readily be derived from conventional pressure measurements by difference approximations. The question is, what use can one make of the ∂P/∂y information, the pressure derivative in the crossline streamer direction provided by the Vy measurement? Due to the wide streamer spacing, ∂P/∂y cannot be derived from the pressure measurements, which are severely undersampled in that direction.
Reconstructing Signals
That is the question the Schlumberger scientist asks himself; then he sees the potential of having access to the ∂P/∂y information. It is related to the theory of discrete sampling, where one of the limitations is the effect called aliasing.
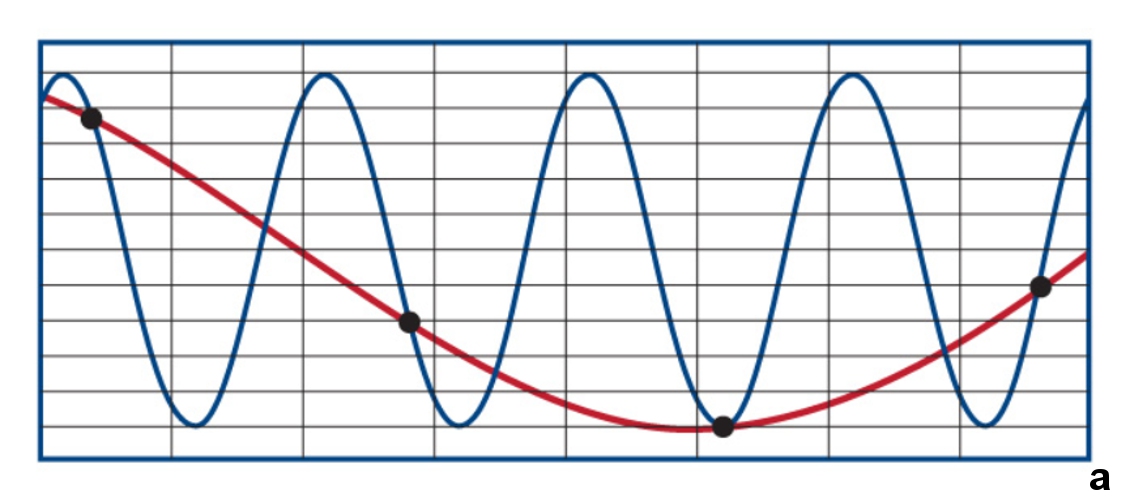
Once more, we step back to basics. An example of aliasing can be seen when watching wagon wheels in old Westerns. Occasionally wheels seem to go backwards when the wagon speeds up, as the rate at which the wheel’s spokes spin approaches the rate of the camera sampler. The same thing happens in seismic data acquisition between the spatial sampler and the spatial signal (or wavefield) being sampled.
Sampling is a fundamental theory in signal processing and its applications. It provides a bridge between continuous bandlimited signals like seismic wavefields and discrete signals (digitally sampled wavefields), allowing the representation, without loss of information, of continuous signals by discrete sequences, which can then be processed digitally. The classic sampling theorem asserts that a bandlimited signal can be perfectly reconstructed from its equally-spaced samples taken at a rate which exceeds twice the highest frequency F present in the signal. A time function which has no frequencies outside the interval [0,F] can be reconstructed exactly from its values at sampling points Δt=1/(2F). This mathematically ideal interpolation result is called Whitaker-Shannon’s (1914, 1949) interpolation formula or sinc interpolation. Shannon referred to the critical sampling interval Δt=1/(2F) as the Nyquist interval corresponding to the band F, in recognition of Nyquist’s discovery (1928) of the fundamental importance of this interval in connection with telegraphy.
Measurement of crossline gradients enables unaliased reconstruction of the seismic wavefield between streamers. The blue waveform represents the actual signal, and the red waveform the reconstructed signal. The figures contrast reconstructed signal with pressure-only measurements in conventional surveys (a) versus pressure and gradients in IsoMetrix surveys (b). (Source: WesternGeco)
Measurement of crossline gradients enables unaliased reconstruction of the seismic wavefield between streamers. The blue waveform represents the actual signal, and the red waveform the reconstructed signal. The figures contrast reconstructed signal with pressure-only measurements in conventional surveys (a) versus pressure and gradients in IsoMetrix surveys (b). (Source: WesternGeco)
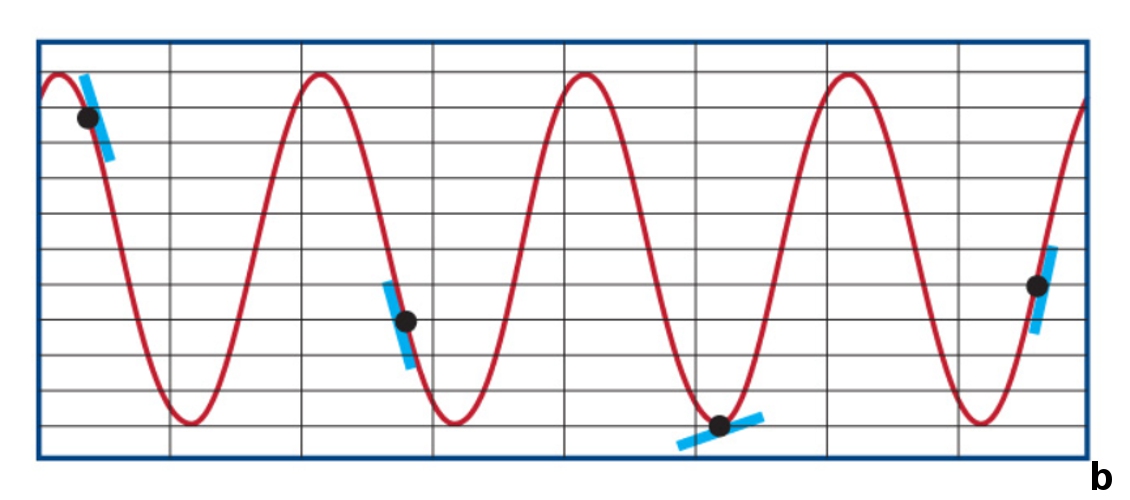
An interesting extension of Shannon’s interpolation formula involves the reconstruction of a bandlimited signal from samples of the signal and its derivatives. Shannon (1949) had remarked that a bandlimited signal could be reconstructed from samples of the signal and its first derivative at half the Nyquist rate, Δt=1/F. lt was Fogel (1955) who first took up Shannon’s suggestion that a function might be reconstructed from its samples together with samples of the derivatives. In Jagerman and Fogel (1959) an explicit formula was given, with a few applications where the sampled derivatives were needed. Their results were generalized by Linden (1959), and Linden and Abramson (1960).
The importance of this result lies in its application. For the geophysicist, the implication is that when both the function and its derivative are sampled at interval Δt=1/(2F), then we can reconstruct signals that have frequencies inside the interval [0,2F].
So what connection did the Schlumberger scientist find between sampling the pressure wavefield and its crossline derivative ∂P/∂y? Access to ∂P/∂y, provided by the Vy measurement, can help interpolation of data between the streamers. More precisely, in between the physical streamers, the data reconstruction theory based on pressure and pressure derivative measurements could be used to predict what the wavefield would be on densely spaced virtual streamers. The Vy measurement would help the geophysicist to ‘read between the receiver lines’.
The first hint of the IsoMetrix solution was published by Johan O. A. Robertsson and co-workers at Schlumberger and WesternGeco in the 2008 October issue of Geophysics. They wrote in their now landmark paper: “Three-component measurements of particle motion would bring significant benefits to towed-marine seismic data if processed in conjunction with the pressure data. A true multicomponent streamer would enable accurate data reconstruction in the crossline direction with cable separations for which pressure only data would be irrecoverably aliased.”
Ideas and Innovation
When ‘The Idea’ was presented internally in Schlumberger, it spurred a lot of new thoughts and innovation as the company put some of its best brains on the challenges ahead. How could this work be extended to improve data reconstruction? The solution was published in two key papers in Geophysics in 2010 by Vassallo, Özbek, Özdemir, Eggenberger, and van Manen. The first discussed multichannel interpolation by matching pursuit using pressure and its crossline gradient, while the second showed the possibility of doing joint interpolation and 3D up/down wavefield separation by generalized matching pursuit.
Reading between the Lines
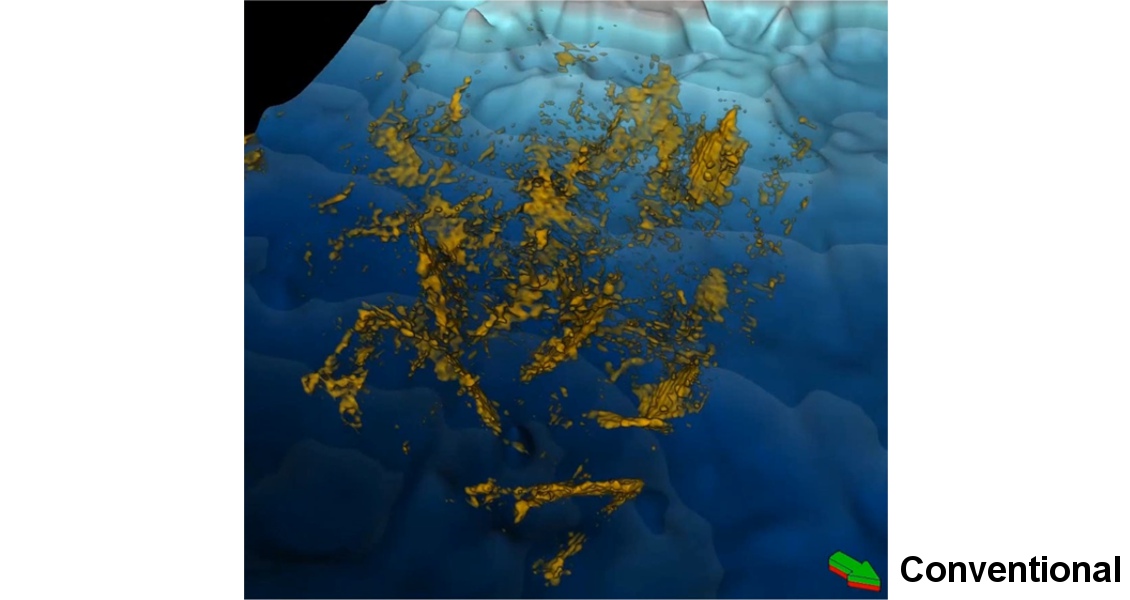
IsoMetrix streamers deploying into the sea. They deliver point-receiver sampling of pressure P from hydrophone and particle velocities Vy and Vz in Y and Z directions from MEMS-based measurements of acceleration. (Source: WesternGeco)Schlumberger immediately realized the potential of the idea, seeing that it effectively removed the conventional measurement gaps between individual streamers to fully capture the returning wavefield in 3D for the first time.
Marine 3D seismic data are typically acquired by a vessel equipped with 8 to 18 streamers, towed 50–100m apart, each 3–8 km long. Using point-receiver recording, the spatial sampling of the data in the inline streamer direction can be as fine as 3.125m, but sampling in the crossline direction is 16–32 times sparser – conventional 3D seismic could better be described as ‘2½ D’. These geometries cannot measure the full 3D wavefield; as we have discussed, the wavefield in the crossline direction can be spatially undersampled, or aliased, and may not allow accurate imaging of the subsurface, especially in complex geology.
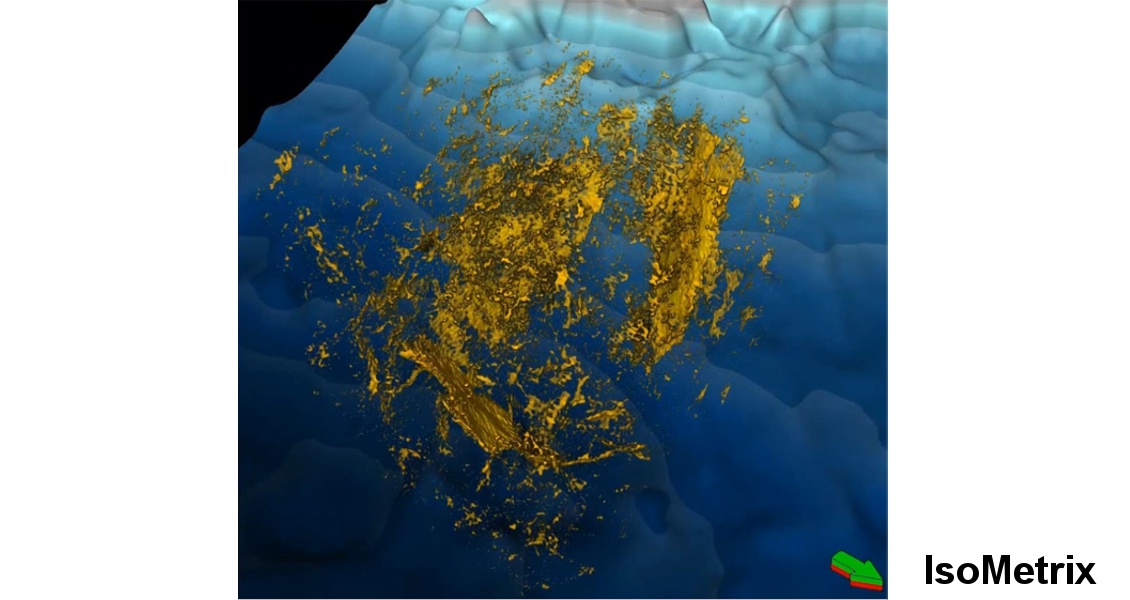
The new data acquisition technology was called IsoMetrix, since the term isometric comes from the Greek for ‘having equal measurement’. Simply stated, IsoMetrix provides a technique to output equal sampling in both crossline and inline directions from measurements of a wavefield that is undersampled in the crossline.
Streamer Noise Mitigation
Another key challenge to be solved was reducing noise in the streamer. The development of a streamer that measures the signal component of particle acceleration was difficult because motion sensors are extremely sensitive to acoustical vibrations within the cable itself.
Schlumberger engineers started developing a new type of streamer, ‘Nessie-6’, which combines traditional hydrophone scalar wave measurements with calibrated point-receiver, multi-measurement accelerometer sensors. These are based on micro-electromechanical system (MEMS) technology, and are used to record the full particle acceleration, giving access to both vertical and horizontal crossline derivatives of pressure. Nessie-6 samples the streamer-borne noise sufficiently for it to be accurately characterized and then removed by digital filtering for both the pressure and acceleration measurements.
Point-receiver measurements enable accurate characterization and subsequent removal of dominant noise modes. This example shows a snapshot of the shape of a 400m streamer section as it is towed through the water (blue represents the top of the streamer and red the bottom). Compressional, transversal and torsional motions all generate noise in the accelerometer measurements. (Source: WesternGeco)
Range of Applications
To summarize, the cornerstone behind the IsoMetrix technology is that when the derivative of the pressure is measured in the crossline direction along with the pressure wavefield itself, on streamers separated by 75–100m, one can reconstruct the pressure wavefield between the cables, creating a set of very densely spaced virtual streamers. In addition, with the measurement of the derivative of the pressure in the vertical direction, the measurements together enable full 3D deghosting to extend the seismic bandwidth. This fine sampling makes the data suitable for a wide range of interpretation and modeling applications, including high-resolution near-surface imaging, deep reservoir characterization, and 4D (time-lapse) reservoir monitoring.
IsoMetrix technology offers a number of benefits compared with conventional acquisition techniques. It enhances acquisition efficiency without compromising sampling or bandwidth. Fine spatial sampling can be achieved with relatively wide streamer separation, maximizing areal productivity. Furthermore, the IsoMetrix deghosting capability means that the entire length of the streamer spread now can be towed deeper than with conventional systems, benefiting from a quieter acquisition environment and extending the weather window.
Acknowledgment:
The authors would like to thank Chris Cunnell of WesternGeco for assistance with this article.
